The following is the final page of an illustrated proof for the
Tough Sudoku of February 20, 2007.
You may need to refer to previous blog pages to understand
this proof. Links to these pages are found to the right, under Sudoku Techniques.
The information on the following blog pages is required to understand this page:
The illustrations of forbidding chains used on this page will share the same key:
- black lines = strong links
- red lines = weak links
- candidates crossed out in red = candidates proven false
Let us set this puzzle to rest!
Still some focus on the 1s
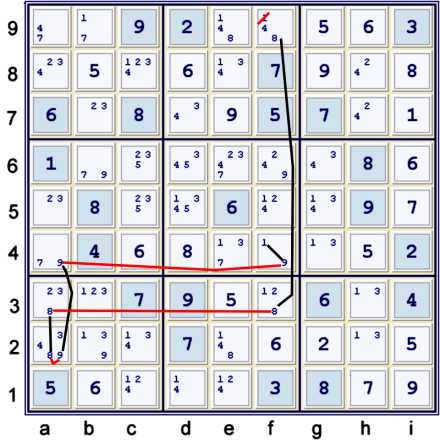
Illustrated above is a relatively easy Forbidding Chain eliminating f9=1. It can be written:
- f9=8 == f3=8 -- a3=8 == a2=8 -- a2=9 == a4=9 -- f4=9 == f4=1 => f9≠1
I saw that this 1 could be eliminated very early on. The only set which has become stronger amongst
those considered is the 8s in column f. Previously, a depth 5 step could have eliminated f9=1 using
the formerly Almost Hidden Pair 68 at f39. Technically, this Hidden Pair still exists, but there
is no need to consider the sixes anymore! At this point, the elimination of the 1 from f9 makes
more sense, as although it does not solve any cells immediately, it allows us to make the next
two relatively easy steps
Still more focus on the 1s
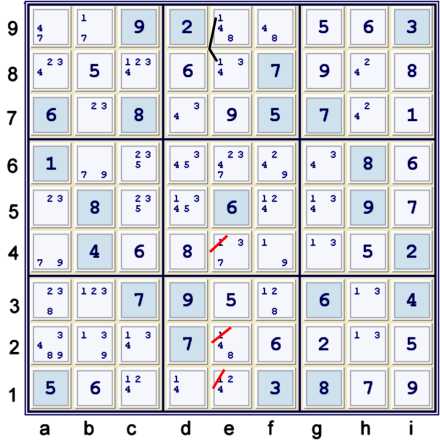
Above Locked 1s at e89 are graphed as a very short Forbidding Chain:
Again, no immediate cell solutions. There are very many paths to take! Hopefully, you can
see many of them.
Easy Depth 4 Forbidding Chain
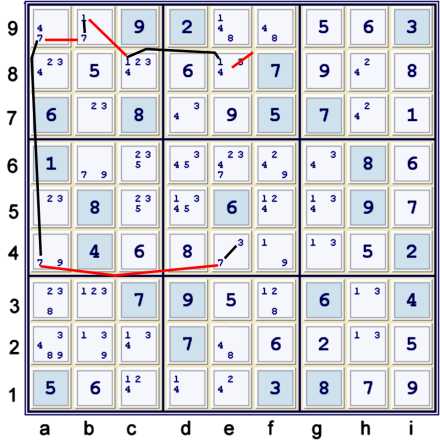
Illustrated above is the following Forbidding Chain:
- e4=3 == e4=7 -- a4=7 == a9=7 -- b9=7 == b9=1 -- c8=1 == e8=1 => e8≠3
Finally, an elimination solves some cells!
- d7 = 3% box
- b7=2, h7=4, h8=2 all % cell (naked singles)
The puzzle is now advanced by
Unique Possibilities to 47 cells solved (UP 47)
The final non-native elimination step
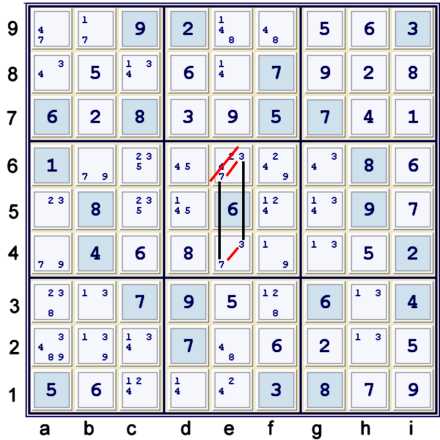
Illustrated above as a Forbidding Chain is Hidden Pair 37 at e46 => e6≠24
One could also use the naked triple 148 at e289 to achieve much the same eliminations, plus
e4≠4. Nevertheless, since the Hidden Pair illustrated above causes e1=2% column, both
lead us to the same place:
- e1 = 2% column
- a3 = 2% row & box
- a5 = 3% cell
- Naked singles to the end!
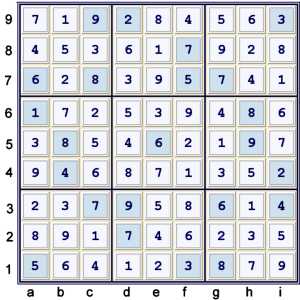
Soltuion ..... Finally!
Proof
A complete proof, pared down to include only those steps deemed required:
- Start at 23 filled - the given puzzle. Unique Possibilities to 27 filled. (UP 27).
- Locked 5's at e23 forbids e46=5
- Locked 5's at gh4 forbids g56i5=5 UP 29
- Hidden pair 29 at g28 forbids g2=13, g8=145
- Locked 3's at h23 forbids h4=3
- Locked 4s at g56 forbids g9=4
- {Hidden pair 719 at a4b6} ==1 a5=7 -- i5=7 == i1=7 --
i1=9 == b1=9 -- b6=9 == a4=9 forbids a4=3
- e12=1 ==1 {b1=6 == d1=6 -- d1=1 ==1 f3=1 -- f4=1
== f4=9 -- a4=9 == a2=9} -- b1=9 == i1=9 -- i7=9 == i7=1 forbids e7=1
- h4=5 == g4=5 -- g4=3 == e4=3 -- e7=3 ==1 {g9=5 == g9=1 --
i7=1 == i7=9 -- e7=9 ==1 e7=4 -- h7=4 ==2
{hidden pair 426}} at h89 forbids h89=5 UP 33
- i1=9 == i1=1 -- h3=1 ==1 {f4=9 == f4=1 -- f3=1 ==1 b3=1
-- b9=1 == b1=7 -- b6=7 == a4=7} -- a4=9 == a2=9 forbids b1=9 UP 38
- h9=6 == h9=4 -- a9=4 == a9=7 -- a4=7 == a4=9 -- a2=9 == b2=9 -- b2=6 == f2=6
forbids f9=6 UP 42
- f9=8 == f3=8 -- a3=8 == a2=8 -- a2=9 == a4=9 -- f4=9 == f4=1 forbids f9=1
- Locked 1's at e89 forbids e124=1
- e4=3 == e7=7 -- a4=7 == a9=7 -- b9=7 == b9=1 -- e9=1 == e8=1 forbids e8=3
UP 46
- Hidden pair 37 at e46 forbids e6=24 UP 81
- Sets: 5(1) + 2(2) + 3(4) + 5 + 2(6) + 7 = 45 (yikes!)
- max depth 7 at step 3.6
- Rating: 5(.01) + 2(.03) + 3(.15) + .31 + 2(.63) + 1.27 = 3.4
A rating of 3.4 qualifies as quite monstrous! Although my rating system is not used by anyone
except me, I think that it gives a reliable accounting of approximate relative difficulty.
Probably the main disadvantage to my rating system is that it does not take into account
the type of step used. Instead, it considers only the depth of each elimination step. I am
always happy to entertain other ideas for ratings. Primarily, I use the ratings to compare
proofs that I may see for a particular puzzle. As such, the ratings are better termed as
proof ratings, and are not really puzzle ratings - although one could say that the lowest
proof rating for a particular puzzle is its rating.
Please do not hesitate to offer alternative proofs for this puzzle. I am quite happy to
find a better way to tackle such difficult puzzles!